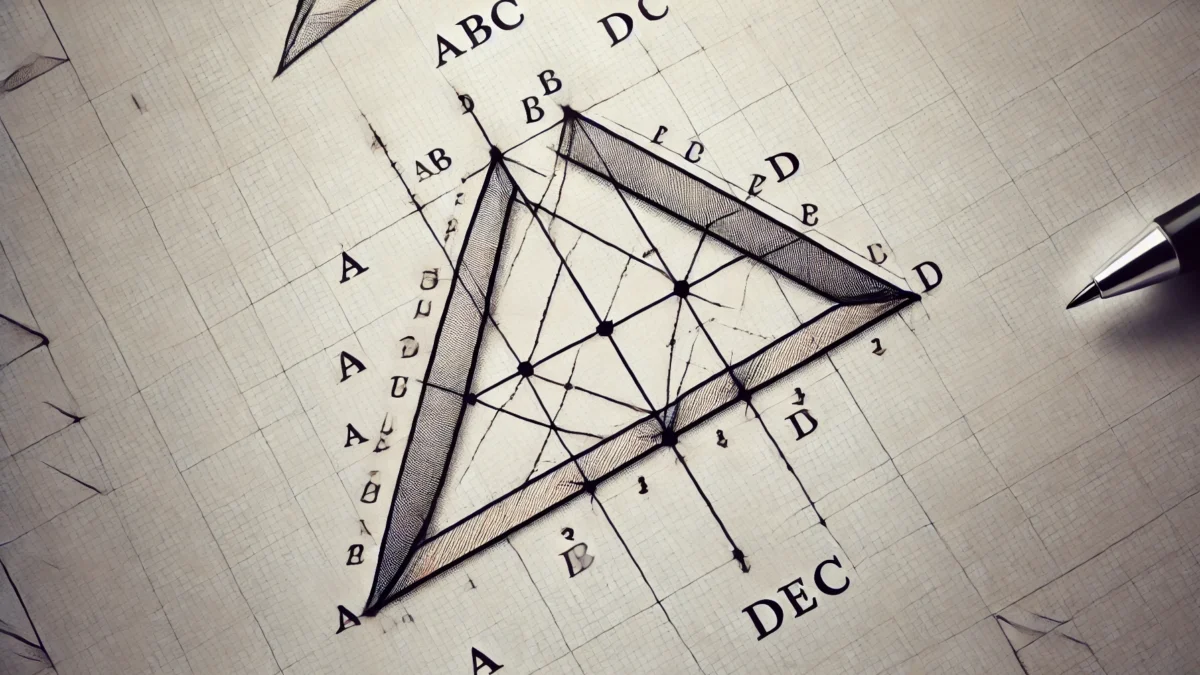
Which Diagram Could Be Used to Prove △ABC ~ △DEC Using Similarity Transformations?
Introduction to Similarity Transformations in Geometry
Similarity transformations are fundamental concepts in geometry that allow us to understand the relationship between two shapes that have the same form but different sizes. These transformations include scaling, rotation, translation, and reflection. When two triangles are similar, their corresponding angles are equal, and their corresponding sides are proportional. This article will explore the specific question: Which diagram could be used to prove △ABC ~ △DEC using similarity transformations? We will delve into the principles of similarity, the types of diagrams that can illustrate these transformations, and step-by-step methods to prove similarity between triangles.
Understanding Similar Triangles: △ABC and △DEC
Before diving into the diagrams, it’s essential to understand what makes two triangles similar. In geometry, two triangles are similar if their corresponding angles are congruent, and their corresponding sides are proportional. For example, if △ABC and △DEC are similar, then:
- ∠A ≅ ∠D
- ∠B ≅ ∠E
- ∠C ≅ ∠C (common angle)
- AB/DE = BC/EC = AC/DC
This proportionality is the foundation of similarity transformations. To prove similarity, we need to identify a diagram that clearly shows these relationships.
Types of Diagrams Used to Prove Triangle Similarity
There are several types of diagrams that can be used to prove triangle similarity. The most common ones include:
- Overlapping Triangles: Diagrams where one triangle is superimposed on another, sharing a common angle or side.
- Parallel Lines with Transversals: Diagrams that show parallel lines intersected by a transversal, creating similar triangles.
- Proportional Sides Diagrams: Diagrams that explicitly label the lengths of sides to show proportionality.
- Angle-Angle (AA) Similarity Diagrams: Diagrams that highlight two pairs of congruent angles in the triangles.
For the specific case of proving △ABC ~ △DEC, an overlapping triangles diagram or a parallel lines diagram is often the most effective.
Overlapping Triangles Diagram: A Visual Proof
An overlapping triangles diagram is one of the most straightforward ways to prove similarity. In this diagram, △ABC and △DEC share a common vertex (usually point C) and overlap in such a way that their corresponding angles and sides can be easily compared.
Steps to Prove Similarity Using Overlapping Triangles:
- Identify the shared angle (e.g., ∠C in both △ABC and △DEC).
- Show that another pair of corresponding angles is congruent (e.g., ∠A ≅ ∠D).
- Use the Angle-Angle (AA) similarity criterion to conclude that the triangles are similar.
This type of diagram is particularly useful because it visually demonstrates the shared angle and the proportional sides.
Parallel Lines and Transversals: Another Approach
Another effective diagram for proving similarity involves parallel lines and transversals. If two triangles are positioned such that their sides are parallel or their bases are aligned with parallel lines, the corresponding angles will be congruent, and the sides will be proportional.
Steps to Prove Similarity Using Parallel Lines:
- Draw two parallel lines and a transversal intersecting them.
- Position △ABC and △DEC such that their sides align with the parallel lines.
- Show that the corresponding angles are congruent due to the parallel lines.
- Use the AA similarity criterion to prove similarity.
This method is particularly useful when dealing with triangles that are not overlapping but still share proportional sides and congruent angles.
Proportional Sides Diagram: Highlighting Side Lengths
A proportional sides diagram explicitly labels the lengths of the sides of both triangles to show that they are proportional. This type of diagram is useful when the angles are not immediately obvious, but the side lengths are known.
Steps to Prove Similarity Using Proportional Sides:
- Measure or label the lengths of the sides of △ABC and △DEC.
- Calculate the ratios of the corresponding sides (e.g., AB/DE, BC/EC, AC/DC).
- Show that all ratios are equal, proving that the sides are proportional.
- Conclude that the triangles are similar using the Side-Side-Side (SSS) similarity criterion.
This approach is particularly useful in practical applications where measurements are available.
Angle-Angle (AA) Similarity Diagram: Focusing on Angles
The Angle-Angle (AA) similarity criterion states that if two angles of one triangle are congruent to two angles of another triangle, the triangles are similar. A diagram focusing on angles can effectively prove similarity.
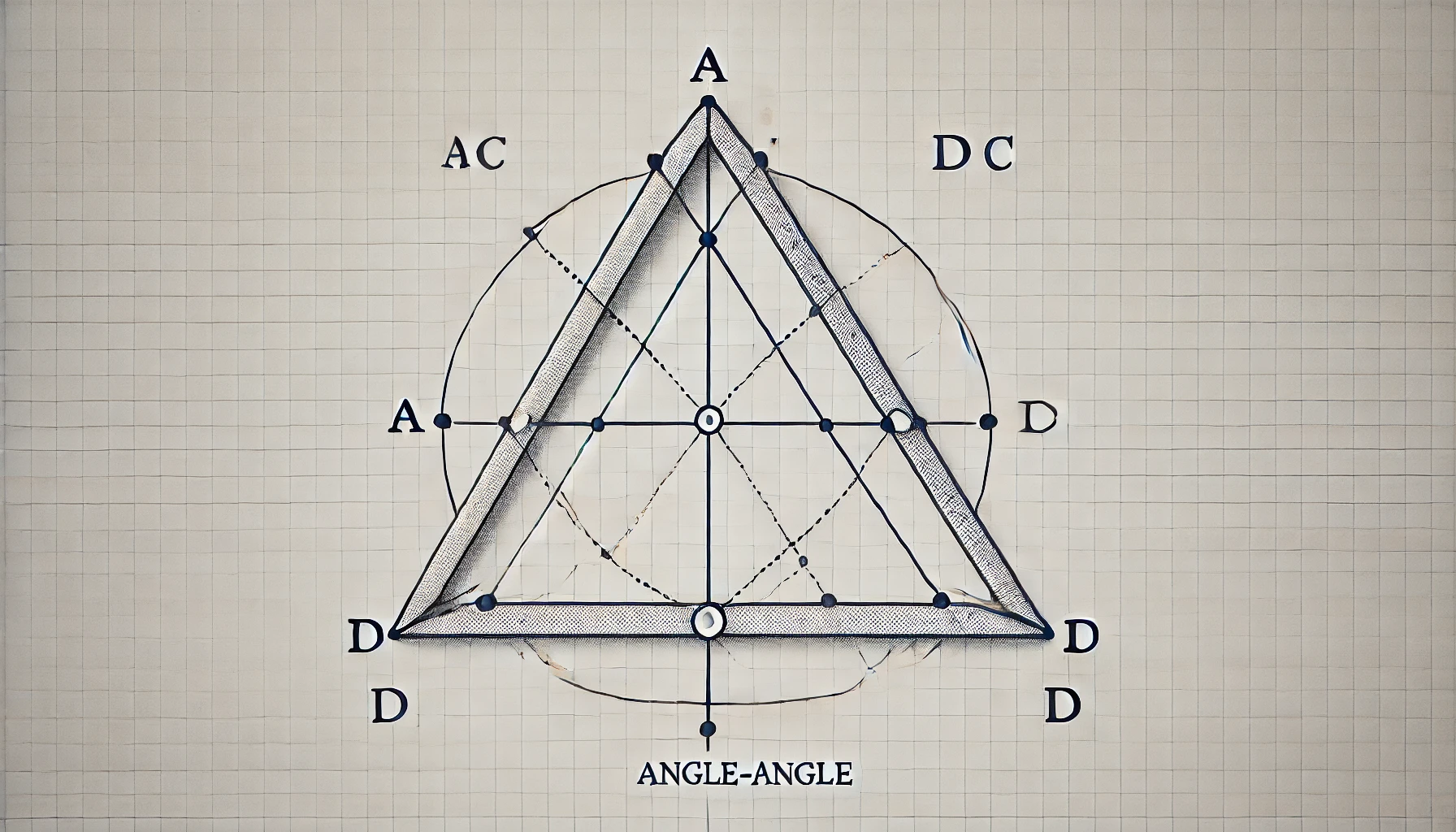
Steps to Prove Similarity Using AA Criterion:
- Identify two pairs of corresponding angles in △ABC and △DEC.
- Show that these angles are congruent (e.g., ∠A ≅ ∠D and ∠B ≅ ∠E).
- Conclude that the triangles are similar based on the AA criterion.
This method is often the simplest and most straightforward way to prove similarity.
Choosing the Right Diagram for △ABC ~ △DEC
When deciding which diagram to use to prove △ABC ~ △DEC, consider the following factors:
- Shared Elements: If the triangles share a common angle or side, an overlapping triangles diagram is ideal.
- Parallel Lines: If the triangles are positioned near parallel lines, a parallel lines diagram may be more effective.
- Known Measurements: If side lengths are known, a proportional sides diagram is useful.
- Angle Information: If angle measurements are available, an AA similarity diagram is the best choice.
For most cases involving △ABC and △DEC, an overlapping triangles diagram or a parallel lines diagram will be the most effective.
Step-by-Step Proof Using an Overlapping Triangles Diagram
Let’s walk through a step-by-step proof of △ABC ~ △DEC using an overlapping triangles diagram.
- Draw the Diagram: Sketch △ABC and △DEC such that they share vertex C and overlap partially.
- Identify Shared Angle: Note that ∠C is common to both triangles.
- Identify Another Pair of Congruent Angles: Show that ∠A ≅ ∠D (or ∠B ≅ ∠E).
- Apply AA Criterion: Since two pairs of corresponding angles are congruent, conclude that △ABC ~ △DEC.
This proof is both simple and visually intuitive, making it a popular choice for demonstrating similarity.
Common Mistakes to Avoid When Proving Similarity
While proving similarity, it’s easy to make mistakes. Here are some common pitfalls to avoid:
- Assuming Similarity Without Proof: Always provide a clear reason (e.g., AA, SSS, SAS) for why the triangles are similar.
- Ignoring Shared Elements: Overlapping triangles often share angles or sides, which can simplify the proof.
- Misidentifying Corresponding Parts: Ensure that corresponding angles and sides are correctly matched.
- Overcomplicating the Diagram: Choose the simplest diagram that clearly illustrates the similarity.
By avoiding these mistakes, you can create a clear and convincing proof.
Practical Applications of Similar Triangles
Understanding how to prove similarity using diagrams has practical applications in fields such as architecture, engineering, and computer graphics. For example:
- Architecture: Scaling models of buildings while maintaining proportions.
- Engineering: Designing mechanical parts that fit together precisely.
- Computer Graphics: Rendering 3D objects with accurate proportions.
Mastering similarity transformations is a valuable skill in these and many other fields.
Read More: Understanding the Chemical Equation for Cellular Respiration
Conclusion: Which Diagram Works Best?
In conclusion, the best diagram to prove △ABC ~ △DEC using similarity transformations depends on the specific information available. Overlapping triangles and parallel lines diagrams are often the most effective, as they clearly illustrate shared angles and proportional sides. By understanding the principles of similarity and choosing the right diagram, you can confidently prove that two triangles are similar. Whether you’re a student, teacher, or professional, mastering these techniques will enhance your geometric problem-solving skills.
